{ A. H. Batten Geodeet 6(30) 1994 6-9 }

Mapping Earth and Space: Astronomy and Geodesy as Partners

A.H. Batten

Dominion Astrophysical Observatory, Herzberg Institute of Astrophysics, 5071, W. Saanich Rd., Victoria, B.C., Canada, V8X 4M6

Teadmised universumi mõõdetest sõltuvad Maa mõõdete teadmisest. Kaugust Päikeseni saame mõõta ainult teades kaugust Maa mõnede üksteisest kaugete punktide vahel. Tähtede kaugusi saame mõõta ainult teades Päikese kaugust. W. Struve andis tähtsa panuse kõigisse nendesse aladesse. Tänapäeva astronoomia tasub oma võlga geodeesiale ülipika baasiga interferomeetria kaudu, mis - rakendatuna kvasaritele ning geodeetilistele tehiskaaslastele - mõõdab maiseid kaugusi niivõrd täpselt, et kontinentide triiv võib osutuda vahenditult vaadeldavaks.

Modern astronomers and modern surveyors follow very different disciplines and acquire very different skills. I doubt if either could do the other's job without undergoing a considerable period of apprenticeship. But this is a new situation, at most two generations old. Early in my own career, I was asked to help a student surveyor who could find no-one else in town to teach him spherical trigonometry. Apparently my efforts were successful: not only did the student qualify, he now has a grown-up son who is an amateur astronomer! Even in my student days, however, the kind of education that left me more familiar with spherical trigonometry than with, say, quantum theory, was somewhat oldfashioned. Nowadays, I suspect that many of our theoretical astrophysicists and cosmologists would be unable to help a budding surveyor with his studies. This is a measure of the change that has overtaken astronomy. Until nearly the end of the nineteenth century, there was little more for the observing astronomer to do than to measure the position of stars on the sky and the apparent brightness of stars. The third dimension of the universe, given by the distances of stars, was lacking altogether until 1838 and could only slowly be delineated during the rest of that century. Only in the last decade of the nineteenth century could we begin to measure changes in the distances of the stars (radial velocities) which could be combined with changes in their position on the sky to give us a picture of the motions in the stellar system as a whole - a picture that led us to understand that that system, or Galaxy, is itself only one of countless millions of similar systems, and enlarged immeasurably humanity's vision of the universe in which it lives. This same enlarging vision attracted so many astronomers that, in some senses unfortunately, they neglected their roots and lost their contact with the Earth and its measurement.

But it was not so in the nineteenth century. The Earth was still the most regular timekeeper known, and astronomers spent much of their time measuring time - by observing the transits of stars across the meridian. Making such observations of stars with transit instruments was not so very different from observing landmarks through a theodolite. The nineteenth-century astronomer was thoroughly expert in just that branch of mathematics - spherical trigonometry - needed to make sense of the surveyors' measurements. Finally, at least in surveys of large areas, the longitudes and latitudes of salient points had to be determined - by astronomical observation. Astronomers simply were the best-equipped people to undertake surveys, and the only other group that governments could call upon for that purpose was their military engineers (often partially trained by astronomers). Wilhelm Struve conceived and participated in many major surveying projects. If I appear to emphasize his contributions to science at the expense of those of Mädler and Öpik - who are also being commemorated at this meeting - it is because I know more about his life and work, even though I have heard Öpik lecture and had a little correspondence with him. Governments supported astronomers in the expectation that the latter would serve them in this matter, as the need arose. Maritime countries were interested in the related problems of determining positions at sea, and they founded observatories whose job was to solve those problems. Even before the French revolution, the astronomers of that country were surveying it and other parts of the globe. During the early nineteenth century that kind of work intensified and Wilhelm Struve plunged into it wholeheartedly. Dr. Dick will discuss the relationship of Struve's work to the wider European efforts in another paper in this symposium. I wish to talk more generally about the relations between astronomy and geodesy.

There is evidence that Struve fully accepted a responsibility to work for the state in this way, in order to justify his requests for purely astronomical instruments such as the Great Refractor here in Tartu (Batten, 1988). On the other hand, he clearly enjoyed the work: he could not have been so successful a surveyor if he had not. But I think he had a third reason for engaging in the work. He was interested in the Earth as an astronomical body: its size and shape could be measured before those of any other such body, and measuring them provided tests of Newton's theory of gravitation. In particular, the figure of the Earth is related to the phenomenon of precession - known since at least the time of Hipparchos (c. 120 B.C.) but not explained until Newton completed the work contained in his Principia. Struve's contemporary and friend, the English astronomer G.B. Airy (1849) said that, if he had lived at the time of Newton, he would have found the explanation of precession to be the most convincing evidence of the truth of the theory of gravitation. The rate of precession depends not only on the masses and distances of the Sun and Moon, but also on the differences of the principal moments of inertia of the Earth. An exact quantitative test of the theory of gravitation, therefore, requires a knowledge of the figure of the Earth. Hence the importance of measuring very long arcs on the surface of the Earth, and, in particular, measuring arcs in both polar and equatorial regions. Struve's arc extended from Hammerfest to the mouth of Danube. He would dearly have loved to extend the measurement through Turkey to Crete, but political relations between the Ottoman and Russian empires made that impossible.

There was still another reason for astronomers to wish to measure the Earth. All distances of objects in space depend on measurements of the relatively nearby objects, which can be made only by observing their parallaxes - a method precisely equivalent to the triangulation used on the surface of the Earth. To measure the distances of Sun, Moon, and planets, we can use places on the surface of the Earth to define our baseline and measure the differences in direction (against the background of distant stars) of the celestial object as observed from the two places. This difference is in the parallax, and to standardize measurements made at very different places on the surface of the Earth, we usually reduce all of them to the equatorial horizontal parallax, that is, the difference in direction of an object as seen on the horizon from a point on the Earth's equator and as seen from the centre of the Earth. It is immediately obvious that to be able to do this we need to know the size and shape of the Earth, and to convert this parallax,π, to a distance, a, we need again to know the Earth's equatorial radius, R, since we have

The principle of the measurement is very simple; the practice is more difficult. Allowances have to be made, for example, for the effect of atmospheric refraction, and for the fact that it is rarely possible to observe from two places at exactly the same time (at least it was difficult to do so before the advent of global wireless telegraphy). Nevertheless, it was fairly easy to measure the equatorial horizontal parallax of the Moon - which is nearly 1°, or about twice the Moon's own apparent diameter. The equatorial horizontal parallax of the Sun, however, is only 8''.8 - and even if it were much larger, measuring it would present many difficulties: there is no fixed point on the Sun's surface, the Sun is too bright to observe directly, and its brightness obscures the background stars with respect to which the measurement is to be made. Fortunately, studies of the motions of the planets, combined with Kepler's and Newton's laws, make it possible to construct a scale model of the solar system: once we have determined one distance within that system, we know them all. So astronomers proceeded by measuring the parallax of one of the planets at a particular time, and inferring from it the distance of the Earth from the Sun.

In Wilhelm Struve's time, the distance from the Earth to the Sun was still only uncertainly known. The first value with anything like modern precision was obtained by G.D. Cassini in the late seventeenth century. He organized simultaneous observations of Mars from widely separated places. His contemporary, Edmond Halley, suggested that transits of Venus could be used to obtain a more accurate value. Observed from widely separated places on the Earth's surface, Venus would appear to transit the Sun's disk by different tracks, and careful observation of those apparent tracks would lead to a determination of the parallax of Venus. The characteristics of the orbits of the Earth and Venus are such that transits of Venus occur in pairs (eight years apart) that are themselves separated by more than a century. Halley's suggestion was sparked by one of the seventeenth century transits - the first known to have been observed - and could not be acted upon until more than halfway through the eighteenth century. Then it provided at least a colourable scientific excuse for some of the European voyages of exploration that led to colonization, and astronomers travelled great distances and endured considerable hardships to try to observe the two transits (Fernie, 1976). The method was less successful than everyone had hoped. It is necessary to observe and to time the first and last instants at which the disk of the planet is seen projected on that of the Sun. Clouds at the crucial times can seriously impair the value of the observations; but, more seriously, the dense cloud in the atmosphere of Venus itself - as we now know - make a clean determination of these times of contact almost impossible. Nevertheless, Struve's close contemporary and one-time friend, J.F. Encke, discussed the results around 1820, and found a parallax of 8''57 - only about 3 per cent too small, and the best value available through Struve's lifetime. Astronomers tried again with the nineteenth-century transits, hoping that their modern instruments and the new inventions of photography and telegraphy would help them to improve the determination, but again the results were disappointing. Wilhelm's son Otto played a prominent role in organizing the Russian efforts to observe those transits.

With the failure of the transit method, astronomers sought other ways to determine the distance

of the Sun. One such was the dynamical way: by study of the planetary motions it is possible to derive the ratio of the mass of the Earth to that of the Sun (mE/mS). Since the quantity, a, that we wish to determine is related to the Earth's orbital period, P, and the constant of gravitation, G, by Kepler's third law:

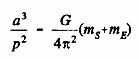
a determination of the ratio mE/mS  is obviously equivalent to a determination of a. (In the above formula, π has its usual geometric meaning, and does not stand for parallax). We note, in passing, that early determinations of G also required surveying techniques, because they depended on measuring the deviation of a plumb line near a mountain, effectively comparing the mass of the mountain with that of the Earth. This kind of measurement was first successfully made in Scotland by Maskelyne in 1775 - less than twenty years before the births of either Struve or Mädler (Davies, 1985). Such measurements were later superseded, of course, by Cavendish's torsion-balance experiment. Returning, however, to the measurement of the distance of the Sun, new prospects were opened towards the end of the nineteenth century by the discovery of the minor planet Eros. This object makes close approaches to the Earth, and therefore has, at such times, a larger parallax than either Mars or Venus, while its star-like image held out hopes that this larger parallax could also be more accurately measured. The classic study of the parallax of Eros was made by Spencer Jones (1941) after the close approach of 1931. His value of the solar parallax (8''79) was indeed of a higher precision than all earlier ones, except one based on measuring the ratio mE/mS  from analysis of the motion of Eros, made twenty years earlier by Noteboom (1921), and which achieved equal precision (0''.001). Unfortunately, the two methods disagreed by much more than their uncertainty, since Noteboom found 8''.799. Nevertheless, Spencer Jones' value was for some time accepted as standard. A fuller discussion of this and other attempts to measure the solar parallax is given by McGuire, Spangler and Wong (1961).

After the Second World War, it became possible to make radar measurements of the distances of some objects in the solar system - particularly of the planet Venus (Price et al., 1959, Evans and Taylor, 1959). Note that we still need to know the shape and size of the Earth, because we are interested in the distance between the centres of the Earth and Venus, and we must observe from some specific point on the surface of the Earth. The radar observations and some of the dynamical calculations made at about the same time, all cluster around a value of the parallax somewhat larger (about 8''.80) than Spencer Jones found, indicating that the Sun is a little closer to us than he believed (but we are talking of less than 200,000 km in about 150 million). There was concern that there might be a systematic error in the radar results. Were we, for example, receiving echoes from an anomalous ionized layer surrounding Venus, thus underestimating the distance of Venus and, therefore, of the Sun? The echoes are now believed to come from the true surface of Venus, and we accept that Spencer Jones' value for the solar parallax was too small. The radar observations are confirmed by measures of perturbations in the motion of spacecraft (McGuire, Morrison and Wong, 1960) and give us the distance of the Sun as accurately as is at present possible, and as accurately as we are likely to need for a long time to come.

All this is something of a digression from the topic of mapping space and, in particular, Strave's contributions to it. But the determination of the distance of the Sun is an important step outwards in our survey of the cosmos. Struve would fully have recognized its importance, even though his own chief contributions were to the step before (mapping the Earth) and the step afterwards (measuring the distances of stars). No baseline on Earth will suffice for the measurement of the parallax of even the nearest stars. The equatorial horizontal parallax of the next star to us after the Sun would be 4''.4·1-6 - well below the abilities of even the most skilled surveyors and astronomers to measure. So the baseline we use is the one traced out by the Earth in its annual journey around the Sun. The angle between the directions of a star as seen from the Earth and from the Sun we call the annual parallax. Even for the nearest star it is only 0''.75 - small, but measurable. As is well known, Struve was one of the first three people to succeed, almost simultaneously, in measuring stellar parallaxes that carried conviction.

This result was of the utmost importance to astronomy. The days were long gone when a successful parallax measurement was needed to convince the world that the Earth revolves around the Sun, but if only the feats of Struve, Bessel, and Henderson had been possible in the time of Galileo, he would have been saved much anguish, and the subsequent history of science, and of the relations between science and religion, might have been very different. At first, measures of stellar parallax proceeded quite slowly; not until photography could be applied to such measurements was any substantial progress made. Even then, there was a strong limit on the distance at which direct measurements of stellar parallax could be considered reliable. The uncertainties of the method, until very recently, were so great that stars more than about six times more distant than Vega - whose parallax Struve measured here in Tartu with the Great Refractor, although he did not publish the measurements until after he had left for Pulkovo - could not yield parallaxes of sufficient precision to be trustworthy. A few exceptions to this statement were stars in clusters, whose parallaxes can be measured differently, and double stars of known orbits, whose components can also be observed spectroscopically. I have myself determined accurate parallaxes in that latter way for some of the doubles that Struve himself discovered - again here in Tartu. We stand on the brink of a revolution in this matter, perhaps as profound as any that Struve himself inaugurated. The European satellite HIPPARCOS, despite the near disaster of its launching four years ago, is successfully measuring thousands of parallaxes with a precision that we could only dream about until recently, and which must amaze and delight the shade of Wilhelm Struve if he is sitting here amongst us.

Nevertheless, up to the present, distances beyond our immediate stellar neighbourhood have had to be estimated by indirect means. I will not go into a discussion of those means, because it takes us beyond the realm in which astronomers and geodesists are partners. They have enabled us, even, to estimate the distances of other galaxies for almost as far as we can see. Again, Struve would share in this triumph - which is built on his own - and yet be amazed. He himself had tried to fathom our own Galaxy in his famous Etudes d'Astronomie stellaire, but did not dare do think that beyond there could be countless other galaxies as big as, or even bigger than, our own. The challenge is still to improve our estimates of distance, which become quite uncertain in the cosmological context. Everyone agrees that the universe is expanding, but there is disagreement by a factor of two about the rate at which it is doing so. The disagreement arises from our uncertainty about the very great distances of other galaxies. But even such uncertain knowledge is a tremendous achievement that Struve and geodesy helped to make possible.

The great vistas of modern cosmology are not, however, the end of my story. To come to that end, we must return to Earth, and plant our feet firmly there, where Struve and others made those important geodetic surveys. But before we make that return journey, we must first introduce some of the most distant objects known - the quasars. They received their name from the fact that their images in even the largest telescopes looked stellar. They were, indeed, believed to be some unusual kind of star until Maarten Schmidt (1963) showed that they were objects with very large Doppler shifts towards the red ends of their spectra. The desire to measure their apparent diameters was one of the stimuli that led to the development of very-long-baseline interferometers, in which two or more radio-telescopes, separated by a great distance, made simultaneous observations of an object that could later be combined electronically to display the interference pattern. I can take some pride in the fact that friends and colleagues in Canada were the first to do this successfully (Broten, et ai., 1967a,b, 1969). Now there are worldwide networks of radio-telescopes engaged in this sort of work. Since the interference pattern observed depends not only on the apparent diameter of the source, but also on the length of the baseline, it follows that changes in the latter quantity can be observed over a sufficiently long period of time. We have, therefore, a method of testing the theory of continental drift. This theory had not even been suggested in Wilhelm Struve's lifetime. If it had been, he probably would have been as sceptical as most of its author's contemporaries were. Nowadays, we nearly all believe in it without question, and, indeed, the evidence that drift occurs is beginning to be available.

The resolution of the initial VLBI measurements was of the order of 10-2 arcseonds, while estimates of the rate of continental drift at that time ranged from 1 cm yr-1 to 15 cm yr-1. At such rates and precision it would have taken some decades of observation to establish the reality of drift. The resolution, of course, is proportional to the wavelength, λ, at which the observations are made, and inversely proportional to the length, D, of the baseline. Since the maximum possible value of D is set by the size of the Earth, and the initial baseline already spanned a continent and an ocean, there was not much scope for improvement to be made by increasing that. Geodetic satellites could, however, be designed for use at appreciably shorter wavelengths (higher frequencies) that were used in the initial measurements and, in fact, plate movements in the range of 5 cm yr-1 to 10 cm yr-1 were already detected some years ago (Ryan and Clark, 1987), although it will doubtless be some decades before a complete picture of what is going on becomes available. Nevertheless, astronomers are beginning to replay the debt that they have owed so long to geodesists. This would have pleased not only Wilhelm Struve, but also, I believe, the other two scientists whose memory we have met here to honour.

References

1. Airy, G.B. 1849, Mem. Roy. Astr. Soc. xx, xxx

2. Batten, A.H. 1988, Resolute and Undertaking Characters: The Lives of Wilhelm and Otto Struve, Reidel, Dordrecht, p. xxx.

3 .Broten, N.W., Legg, T.H., Locke, J.L., McLeish, C.W., Richards, R.S., Chisholm, R.M., Gush, H.P., Yen, J.L. and Galt, J.A. 1967a, Nature, 215, 38.

4. Broten, N.W., Clarke, R.W., Legg, T.H., Locke, J.L., McLeish, C.W., Richards, R.S., Yen, J.L., Chisholm, R.W., and Galt J.A. 1967b, Nature, 216, 44.

5. Broten, N.W., Clarke, R.W., Legg, T.H., Locke, J.L., Galt, J.A., Yen, J.L. and Chisholm, R.M. 1969, Mon. Not. Roy. Astron. Soc., 146, 313.

6. Davies, R.D. 1985, Quart. J. Roy. Astron. Soc., 26, 289.

7. Evans, J.V. and Taylor, G.N. 1959, Nature, 184, 1358.

8. Fernie, J.D. 1976, The Whisper and the Vision, Clarke Irwin & Co. Ltd., Toronto.

9. Jones, H.S. 1941, Mem. Roy. Astron. Soc., 66, pt ?, 11.

10. McGuire, J.B., Morrison, D.D. and Wong, L. 1960,        Astr. J., 54, 493.

11. McGuire, J.B., Spangler, E.R. and Wong, L. 1961,        Scientific American, 204, No. 4, 64

12. Noteboom, E. 1921, Astr. Nachr., 214, 153.

13. Price, R., Green, P.E.jun., Goblick, T.J., Kingston, R.H., Kraft, L.G.jun., Pettengill, G.H., Silver, R. and Smith, W.B. 1959, Science, 129, 751.

14. Ryan, J.W. and Clark, T.A. 1987, in: The Impact of VLBI on Astrophysics and Geophysics (LAU Symp. No. 129), eds. M.J. Reid and J.M. Moran, Kluwer, Dordrecht, 339.

15. Schmidt, M. 1963, Nature, 197, 1040.